In this blog, Pip Huyton, a mathematics consultant, explores the importance of visualising 3D shapes within spatial reasoning at KS1 and KS2. So, let’s jump right in (in a true mathematician’s way) by looking at the data!
What does the data suggest – Why is the visualising of 3D shapes important?
When looking at the data of the national KS2 SATs 2024 (outcomes by question), the eye is always drawn to the pieces of data that ‘buck the trend’. I am not aware that the data is expected to rigidly align with a statistical ‘line of best fit’ but is used here as a tool to assist when reviewing.
Look at Question 13 on Paper 2, a mid-paper question. What does the data show? Less than 50% of the cohort submitted a correct answer. 11% less than might be anticipated using the ‘trend line’ of the graph below. Question 13 is on the topic of 3-D shape as is Question 23 on Paper 3, which received 23% of the cohort answering correctly, the lowest percentage and furthest below the trend line for any question on the paper.
Whilst the circled red data points are questions focusing on 3-D shape only, it is worth noting all Geometry questions on Paper 2 (questions’ 1,13, 21 and 26) and on Paper 3 (questions’ 8, 10 ,20 and 23) fall below the ‘line of best fit’ in the graphs! So, let’s take a look at some of the questions in more detail.
Examples of the 3D Shape SATS Questions (2024)
Here are the questions. Let’s take a look at them and look at how the skill of visualising 3D shapes could help.
These two 3-D Shape questions primarily relate to National Curriculum content from Years 1-3 of the Programmes of Study for Mathematics (2013), with the KS2 expectation for children to recall mathematical subject knowledge and reason using the knowledge.
A key requirement for children to answer these questions would be to have a visual recall (visualising 3D shapes), or mental image of the shapes. Based on the outcomes detailed above, it would suggest a significant proportion of children do not have a working command of this knowledge/skill and find visualising 3D shapes difficult. Insecure foundations are likely to impact on children when working at KS3/KS4.
How can teachers help children with visualising 3D shapes
Children need to be provided with the opportunity to develop a visual mental recall of 3-D Shapes.
Children should actively play and explore in Mathematics at every stage – not just in the EYFS and KS1. Play allows children to observe, make sense of, and provide a concrete example to help communicate their thinking and understanding. Play is an essential part of children having the opportunity to develop the desirable mathematical behaviours of young mathematicians. Children need to be able to:
- conjecture
- predict
- justify
- compare and contrast
- challenge another’s thinking through asking questions and discussion
- generalise
Below is an image explaining each of the above in a bit more detail.

Visualising 3D shapes in Mathematics
When children build a strong mental image of 3D shapes, they rely less on memorising separate facts. Visualisation empowers the child to identify multiple facts, answer questions and reason solutions to problems.
Talk in mathematics should not be seen simply as a rehearsal in class of the vocabulary of mathematics… It should extend to high-quality discussion that develops children’s logic, reasoning and deduction skills, and underpins all mathematical learning activity. The ultimate goal is to develop mathematical understanding – comprehension of mathematical ideas and applications.
The Williams Report (2008)
What is the role of the teacher
How can teachers support children in this area? The role of the teacher is to:
- provide learning opportunities/tasks for the children’s mathematical habits to be developed
- probe children’s thinking with appropriate questions
- introduce and refine mathematical language whilst allowing children to talk freely in a non-scripted manner
Providing support with 3-D Manipulatives
Provide the children with a suitable range of 3-D shapes to touch and hold, connect and separate, compare and sort. By doing these things, children will improve their visualising of 3D shapes.
An example: A set of ten different 3-D shapes; cube, triangular prism, rectangular prism, hexagonal prism, triangular pyramid, square pyramid, sphere, hemisphere, cone and cylinder. The 3D shapes are in 4 different colours. This provides opportunities for children to explore the content required for primary school learning. Note: this set, unlike many others, includes a hexagonal prism. This encourages children to think about 3-D shapes with polygons of more than 4 sides.
Examples of tasks to support learning
The following tasks are intended for children to do in pairs or small groups. Each task can be adapted for the age and/or prior knowledge of the children when selecting the 3D shapes to be used. The purpose of the tasks are to:
- encourage children to talk/discuss as they touch and play with the 3-D shapes
- gather an insight into the key features of the shapes
- support the development of visualising shapes – which is hard to achieve from looking at only diagrams
1. Feely bag
This task helps children to develop and use their sensory and oracy skills to describe 3-D shapes.
- A selection of 3-D shapes is placed in a non-transparent bag.
- One child puts their hand in the bag, selects one shape without removing the shape from the bag or looking inside.
- Using touch alone, the child must describe the selected shape to their partner/s, one detail at a time.
- Partner/s suggest the identity of the shape either by naming it or pointing to what they think the shape is from a separate set of shapes.
- Once children think they have identified the shape, it can be removed from the bag to check.
- Repeat the task with another child making the shape selection.
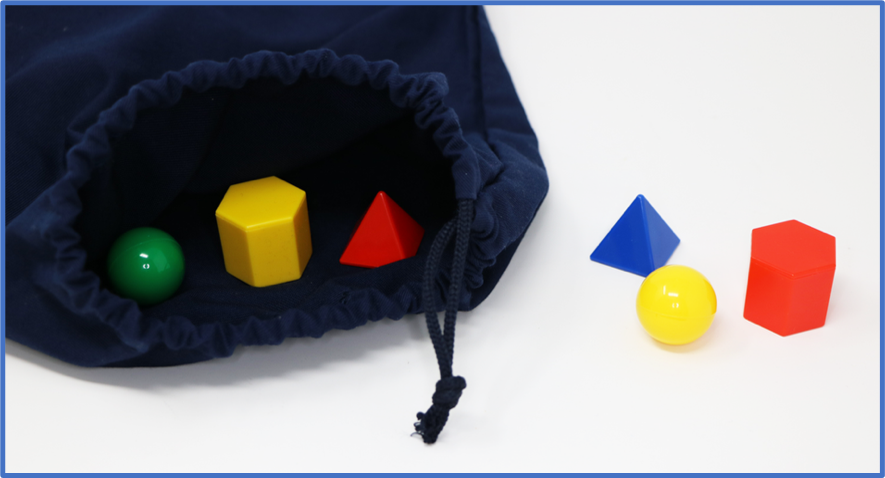
Feely bag with 3-D shapes inside
Key questions:
- What vocabulary/descriptions are most helpful?
- How many pieces of information do the children need to identify the shape correctly?
2. Sorting
This task helps children to identify and categorise the key features of the 3-D shapes
- A selection of 3-D shapes is placed on a large piece of card.
- Working in pairs or a small group of children, ask the children to sort the physical shapes into 2 groups, agreeing the sort criteria.
- Allow children to describe the criteria using their own description which can be refined to mathematical language. e.g. Shapes with a ‘Rounded surface’ (curved surface) and shapes without.
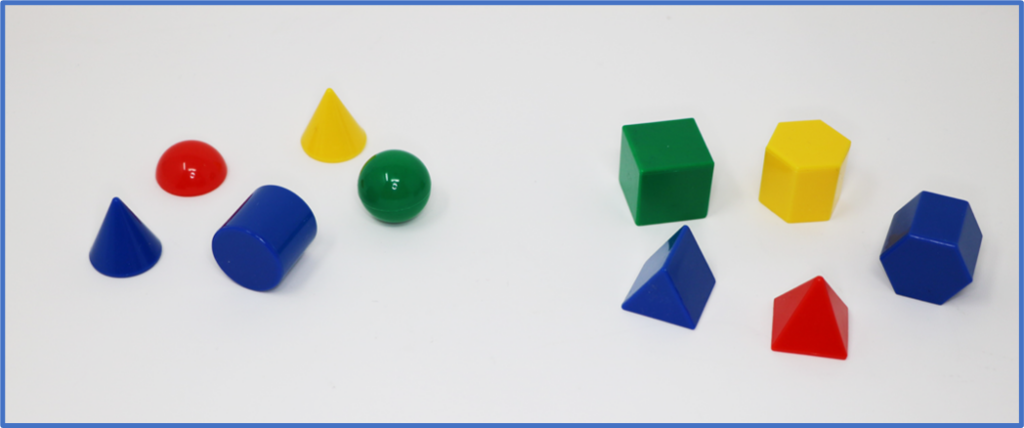
Shapes sorted into 2 groups
Key questions:
- How do you decide which group a shape is to be placed in (sorting criteria)?
- How can you sort the same selection of shapes in a different way?
- How are your sorted groups of shapes the same or different to another pair/groups? What criteria have they used to sort the shapes?
The task could be repeated using shapes with some common elements (Venn Diagram), or shapes sorted into 3 separate groups, or 2 dimensional shapes could be included.
3. What’s my shape?
This task encourages children to ask questions and in doing so develop, refine and practise their skills in precision of questioning and use of mathematical language.
- Two children face each other with a white board, or similar, acting as a barrier between them. Ensure neither can see what is on the opposite side. Alternatively, children could sit back to back.
- A child selects a 3-D shape and hides it behind the barrier from their partner.
- The partner must ask questions to identify the hidden shape.
- Initially, allow questions to be open. As the task is repeated, it can be more challenging if the child with the shape can only respond with yes or no to questions.

Key questions:
Questions the child could ask their partner to help them identify the shape include: Are all the faces the same shape?, How many faces does the shape have? Does it have a ‘pointy’ end?
Examples of refinements that can be made to a question/answer (supported by the teacher) could include, What is the mathematical term for a ‘corner’? Is the end at the top or the bottom – does it matter, is the orientation important? Are some questions more helpful/informative than others? – make a list of ‘good’ questions
4. WODB – Which one doesn’t belong?
This task helps children practise and strengthen their visualising skills, and critical and creative thinking with reasoning.
- The names of different 3-D shapes/objects are placed on the 4 sections of the card.
- Children will need to visualise the four shapes/objects, and use their knowledge of the shapes to reason which they consider to be the ‘odd one out’ based on the attributes of the shapes.
- The choice of shapes/objects should not lead to there being only one correct answer.
- Encourage children to think creatively when looking for attributes.
- A collection of cards can be made for future use. These could include landmarks etc which link with a recent humanities topic.
E.g. WODB – possible reasons for selection

Football because…it is made up of hexagons and pentagons and/or it has a curved surface.
Square because…it is 2-D and/or it has 4 sides (not edges).
Cube because…all faces are equal in area and/or a regular polyhedron (3-D shape).
Pyramid because…it has triangular faces and/or it has a point (apex) when its base is on the table.
Key questions:
- Do all pyramids have the same shape base?
- Are all footballs made from Hexagons and Pentagons?
- What’s the difference between a pyramid and a prism?
- Which 3-D shapes always have the same shape face at either end?
- What other shapes have an apex?
For further information
If you would like further information on how visualisation fits into the curriculum, Pip has included this handy download. Click here or on the image to see full document.
If you would like to read more of Pip’s work, why not take a look at other books she has written:
Build-it Say-it Write-it Understanding the concepts of Perimeter and Area using Manipulatives
ISBN 978-1-912185-32-0 available via Association of Teachers of Mathematics
KS1 Assessment Tasks Not Tests
Freely available via Association of Teachers of Mathematics and Mathematical Association websites
If you would like to reach out to Pip, contact her by email – pip@accomplisheducation.com
With thanks to Pip Huyton for writing this blog.
Pip Huyton is a mathematics consultant working in both the primary and secondary sectors. She is a trainer, adviser and facilitator, often in a bespoke school improvement capacity. Interests include curriculum development, the use of manipulatives for supporting children developing conceptual understanding and effective use of dynamic technology for learning.























